Hmmm... where to start, where to start...
Well, this unit has been fairly interesting because of its relation with the previous unit. Now I can begin to see how everything in Calculus ties together. I have enjoyed this unit quite a bit because it has a decent amount of material that relates to Physics...
As well, it is fairly straight forward with the math, not a lot of outside the box thinking...
A key thing to remember is:
- There is more than one way to find the integral of a function
1. Using the formula: lim(n-->∞) [ f(x1)•∂x1 + f(x2)•∂x2 + ... f(xn)•∂xn ]
2. The Riesum Program in our Calculators:
LEFT: (left side of the interval)
RIGHT:(right side of the interval)
X CHOICE: (0=Left Estimate)
(0.5=Midpoint Estimate)
(1=Right Estimate)
N: (number of sub intervals)
3. "fnInt" function in our calculators: fnInt(Y1, X, min, max)
4. the function "∫ ƒ(x)∂x" in the 2nd Trace (Calc.) Menu
5. Calculate it Manually: LEFT ESTIMATE: Sum of all "ƒ(x)"s, minus the last "ƒ(x)" then multiplied by ∂x.
RIGHT ESTIMATE: Sum of all "ƒ(x)"s, minus the first "ƒ(x)" then multiplied by ∂x.
TRAPEZOID SUM 1: Sum of the first "ƒ(x)", the last "ƒ(x)", and (2 • all the "ƒ(x)"s in between) Then multiplied by ∂x. Finally divide by 2.
TRAPEZOID SUM 2: (LEFT ESTIMATE + RIGHT ESTIMATE) /2
Well, that's pretty much all for my BOB, good luck tomorrow... and:
Wednesday, October 31, 2007
BOB
Well, this unit about definite integrals was an easy one for me. I felt like I understand all the concepts and do all the homework without stressing too much or even asking my sister. I am really confident that this test shouldn't be too hard, but as for precautionary measures, I will just make sure that i study well for tomorrows test. I hope that everyone will get a good mark on tomorrow's test. Study well and good luck.
BOBling
Hello everyone, and it is time for our second bob for the ap calculus class of 07-08. It is too bad I could not attend school today as I attended a United Way Leadership convention/workshop spanning the entire school day instead. Though, I have been present for the majority of our most recent unit, and must agree with Graeme when I say that I too feel very confident going into this unit's test. I believe that the test shouldn't be too much of a challenge, and that most of our class should handle the test with remarkable ease tomorrow. I can't recall any tribulations I encountered throughout this unit or in the pre-test, and the exercises found in our textbook weren't exactly that difficult so this test should not present too many unfamiliar questions. All I can say is that I'm not worrying too much about this test, but am definitely excited to begin my favorite unit in calculus--differentiation. Well, I guess that's really all I can say for this BOB, though the test doesn't seem particularly challenging (though it is Mr. K) I probably will still further my preparation for the test by completing more exercises or questions pertaining to this unit as should everyone in the class. I wish everyone, along with myself, good luck on tomorrow's test! Good night everyone!
Bobbin'
Well I have to say I seem to get this unit fairly well. Enough so that I feel confident that I will get a good mark on the test. Especially after that pretest, felt pretty good throughout that. Must say this has been far easier than grade 12 so far. I wish you all luck on the test!
BOB 2
Well it's time to bob for the second time now. I have found this unit much lighter and easier then the second one. I feel much better about myself going into this test. I hope to do well on the test and wish luck to everyone else. I pretty much understand everything in the unit and had fun with integrals. Now it's just a matter of applying it on the test! Well good luck to you all and do well. Bye!
Tuesday, October 30, 2007
13-0-13 V. 2
Hello!
It seems I find myself blogging on blogging again! This unit went by pretty quickly, especially since there were only 4 parts in the textbook in Chapter 3. I was actually quite surprised that I have been understanding and getting most of the questions because usually I'm quite confused. I've been doing all right in the group work so hopefully I will be able to do well on the test. The pretest was all right I got most of it right which is good and things cleared up when we had discussed things with our group and in our MEGAGROUP during the fire drill. Each time I got something right I got super excited! I really hope that I do well on the test, actually I hope we all do well. Good luck to everyone!
It seems I find myself blogging on blogging again! This unit went by pretty quickly, especially since there were only 4 parts in the textbook in Chapter 3. I was actually quite surprised that I have been understanding and getting most of the questions because usually I'm quite confused. I've been doing all right in the group work so hopefully I will be able to do well on the test. The pretest was all right I got most of it right which is good and things cleared up when we had discussed things with our group and in our MEGAGROUP during the fire drill. Each time I got something right I got super excited! I really hope that I do well on the test, actually I hope we all do well. Good luck to everyone!
Pre-Test Scribe
Hello everyone. I’m Robert and I am the scribe for today.
Today’s class started off with a discussion about blogging in other classes other than a math class. We talked about how important blogging was in our calculus class and compared to other classes. Feel free to give your opinions about blogging in your school or classroom in the comments to this post!
After that very interesting discussion on blogging, Mr. Kuropatwa told us about two other very cool tools to put in our tool box. Here they are:
Sketchcast.com
&
http://www.blogger.com/www.scribd.com
Feel free to explore!
Then we moved onto the pre-test. To see what the pre-test looks like, just look to the slides for October 30, 2007.
Today’s class started off with a discussion about blogging in other classes other than a math class. We talked about how important blogging was in our calculus class and compared to other classes. Feel free to give your opinions about blogging in your school or classroom in the comments to this post!
After that very interesting discussion on blogging, Mr. Kuropatwa told us about two other very cool tools to put in our tool box. Here they are:
Sketchcast.com
&
http://www.blogger.com/www.scribd.com
Feel free to explore!
Then we moved onto the pre-test. To see what the pre-test looks like, just look to the slides for October 30, 2007.
To end off the class we had a fire drill.
Well that's it guys, and the next scribe is MrSiwWy.
Monday, October 29, 2007
Scribe (O.o)
Hello, I'm Tim-Math-y and I will be your scribe for today's class lessons. Today, we started off class by going over the requirements for scribe posts.
Remember that you are required to label your posts accordingly, especially your scribe posts. You are required to label your scribes with your name, unit, and most importantly, 'scribe'. Without labelling your scribe with 'scribe' is equivalent to not placing your name on your work; you will not receive credit due to completion on the scribelist (If you have not already fixed your labels, you should as soon as possible).
Next, we talked about del.icio.us accounts. If you have not already signed up for one such account, it is required as soon as possible. Remember to check out other tags in math, calculus, and the such for sites that other people found extremely useful. These sites will be helpful in contributing aid in our learning outside of the class.
After the brief run overs of these topics, we continued onto learning MATH! Today, we had a workshop to prepare us for a Pretest tomorrow and a Test on Thursday.
 1.a) For this question, we simply solved the lower and upper estimates using our calculators. We placed the equation f(x) = 4x - x^2 into y1 and used our RIESUM program to solve from the interval of [0,1]. Our solutions were L(4) = 1.28125 and U(4) = 2.03125.
1.a) For this question, we simply solved the lower and upper estimates using our calculators. We placed the equation f(x) = 4x - x^2 into y1 and used our RIESUM program to solve from the interval of [0,1]. Our solutions were L(4) = 1.28125 and U(4) = 2.03125.
1.b) We simply subtract the lower estimate from the upper estimate.
1.c) Here, we sketched 4 sub intervals on the interval of [0,1] of f(x). Here we geometrically sketched the upper and lower estimates to represent U(4) - L(4). The left sketch is incorrectly scaled whereas the right sketch is a correct drawing.
1.d) We used the equation: Error = |f(b) - f(a)| x ((b-a)/n) to solve. Here, 'b' is equal to 1 where 'a' is equal to 0.
 2.) Here, we analysed the table to find out how we could estimate the integral on the interval of [-2,4]. Considering the fact that we need 3 sub intervals, we found that from (-2) -> (4) was 6 and so we could easily divide the information into 3 sub intervals. Here, we found the midpoint of 1.98 and 2.04 which was 1.03, midpoint of 2.04 and 9.64 which was 5.74, and the midpoint of 9.64 and 26.29 which was 16.82. We then added the three numbers and multiplied the sum by 2, because the subintervals maintained the length of 2. We found the result of 47.18 (Credit goes to Craig's group)
2.) Here, we analysed the table to find out how we could estimate the integral on the interval of [-2,4]. Considering the fact that we need 3 sub intervals, we found that from (-2) -> (4) was 6 and so we could easily divide the information into 3 sub intervals. Here, we found the midpoint of 1.98 and 2.04 which was 1.03, midpoint of 2.04 and 9.64 which was 5.74, and the midpoint of 9.64 and 26.29 which was 16.82. We then added the three numbers and multiplied the sum by 2, because the subintervals maintained the length of 2. We found the result of 47.18 (Credit goes to Craig's group)
Another group attempted something different. They plotted the points onto the statplot using their calculators and simulated a similar quadratic graph. The solution was fairly close (46.5248) however despite the 99% accuracy, the answer was incorrect as it was not as accurate using the information given.
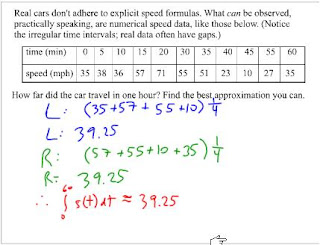 3.) Here, Chris' group attempted to solve the problem. He came up with the idea to use four subintervals, seeing that the information given could not be broken up properly into 5s nor 10s and so he broke them into groups of 15s. This brought forth much sense. However, the answer was not the best approximation as he did not use all the information given.
3.) Here, Chris' group attempted to solve the problem. He came up with the idea to use four subintervals, seeing that the information given could not be broken up properly into 5s nor 10s and so he broke them into groups of 15s. This brought forth much sense. However, the answer was not the best approximation as he did not use all the information given.


Here, Craig's group again brought forth a solution. They used the RIESUM formula to find the best approximations on seperate intervals (the riesum finds the sum of the lower and upper estimates and divides it by 2). The intervals were: [0,20] which had intervals of 5 minutes, [20,30] which had an interval of 10 minutes, [30,45] which had intervals of 5 minutes, [45,55] which had an interval of 10 minutes, and [55,60] which had an interval of 5 minutes.
Adding together all the solutions, he found a more accurate approximation because he used all of the information given, despite the extreme excess of work compared to Chris' method.
Remember that you are required to label your posts accordingly, especially your scribe posts. You are required to label your scribes with your name, unit, and most importantly, 'scribe'. Without labelling your scribe with 'scribe' is equivalent to not placing your name on your work; you will not receive credit due to completion on the scribelist (If you have not already fixed your labels, you should as soon as possible).
Next, we talked about del.icio.us accounts. If you have not already signed up for one such account, it is required as soon as possible. Remember to check out other tags in math, calculus, and the such for sites that other people found extremely useful. These sites will be helpful in contributing aid in our learning outside of the class.
After the brief run overs of these topics, we continued onto learning MATH! Today, we had a workshop to prepare us for a Pretest tomorrow and a Test on Thursday.
Workshop
 1.a) For this question, we simply solved the lower and upper estimates using our calculators. We placed the equation f(x) = 4x - x^2 into y1 and used our RIESUM program to solve from the interval of [0,1]. Our solutions were L(4) = 1.28125 and U(4) = 2.03125.
1.a) For this question, we simply solved the lower and upper estimates using our calculators. We placed the equation f(x) = 4x - x^2 into y1 and used our RIESUM program to solve from the interval of [0,1]. Our solutions were L(4) = 1.28125 and U(4) = 2.03125.1.b) We simply subtract the lower estimate from the upper estimate.
1.c) Here, we sketched 4 sub intervals on the interval of [0,1] of f(x). Here we geometrically sketched the upper and lower estimates to represent U(4) - L(4). The left sketch is incorrectly scaled whereas the right sketch is a correct drawing.
1.d) We used the equation: Error = |f(b) - f(a)| x ((b-a)/n) to solve. Here, 'b' is equal to 1 where 'a' is equal to 0.
 2.) Here, we analysed the table to find out how we could estimate the integral on the interval of [-2,4]. Considering the fact that we need 3 sub intervals, we found that from (-2) -> (4) was 6 and so we could easily divide the information into 3 sub intervals. Here, we found the midpoint of 1.98 and 2.04 which was 1.03, midpoint of 2.04 and 9.64 which was 5.74, and the midpoint of 9.64 and 26.29 which was 16.82. We then added the three numbers and multiplied the sum by 2, because the subintervals maintained the length of 2. We found the result of 47.18 (Credit goes to Craig's group)
2.) Here, we analysed the table to find out how we could estimate the integral on the interval of [-2,4]. Considering the fact that we need 3 sub intervals, we found that from (-2) -> (4) was 6 and so we could easily divide the information into 3 sub intervals. Here, we found the midpoint of 1.98 and 2.04 which was 1.03, midpoint of 2.04 and 9.64 which was 5.74, and the midpoint of 9.64 and 26.29 which was 16.82. We then added the three numbers and multiplied the sum by 2, because the subintervals maintained the length of 2. We found the result of 47.18 (Credit goes to Craig's group)Another group attempted something different. They plotted the points onto the statplot using their calculators and simulated a similar quadratic graph. The solution was fairly close (46.5248) however despite the 99% accuracy, the answer was incorrect as it was not as accurate using the information given.
 3.) Here, Chris' group attempted to solve the problem. He came up with the idea to use four subintervals, seeing that the information given could not be broken up properly into 5s nor 10s and so he broke them into groups of 15s. This brought forth much sense. However, the answer was not the best approximation as he did not use all the information given.
3.) Here, Chris' group attempted to solve the problem. He came up with the idea to use four subintervals, seeing that the information given could not be broken up properly into 5s nor 10s and so he broke them into groups of 15s. This brought forth much sense. However, the answer was not the best approximation as he did not use all the information given.

Here, Craig's group again brought forth a solution. They used the RIESUM formula to find the best approximations on seperate intervals (the riesum finds the sum of the lower and upper estimates and divides it by 2). The intervals were: [0,20] which had intervals of 5 minutes, [20,30] which had an interval of 10 minutes, [30,45] which had intervals of 5 minutes, [45,55] which had an interval of 10 minutes, and [55,60] which had an interval of 5 minutes.
Adding together all the solutions, he found a more accurate approximation because he used all of the information given, despite the extreme excess of work compared to Chris' method.
End of Workshop
Word of the Day: Ginkgo - a herbal remedy derived from Jap/Chi tree to improve mental function and circulation.
Tomorrow's Scribe: aichelle s. Show them a nice scribe =)
Reminders: Tomorrow Pretest, Workshop Wednesday, Test Thursday
Have a great night everyone! Good luck on the pretest and test this week! Don't forget to BOB also! Good night!
Word of the Day: Ginkgo - a herbal remedy derived from Jap/Chi tree to improve mental function and circulation.
Tomorrow's Scribe: aichelle s. Show them a nice scribe =)
Reminders: Tomorrow Pretest, Workshop Wednesday, Test Thursday
Have a great night everyone! Good luck on the pretest and test this week! Don't forget to BOB also! Good night!
Saturday, October 27, 2007
Scribe
Hello. I'm Sandy and I'll be your scribe for this weekend. Friday's class started off like every other class we have... Graeme putting the Word of the Day on the board. Friday's word was...
Bacchanalia: "Generally just a wine festival but really is a wine festival in honor of Dionysus. (Greek god of horses and drunkenness) (Poseidon is lord of horses)."
Del.icio.us
If you don't have a Del.icio.us account yet, you should make one!
Http://del.icio.us/
Your username should not include your last name. If you're having trouble you can add the class blog and your first name.
Example: Sandyapcal07
Assignment: Find one link for each unit that we've studied and add it to Del.icio.us. It can be anything, but at least put some effort into it and don't just pick the first website you see. So far you have two links to add, Pre Calculus and Derivatives.
Screen-O-Matic
This is a program on the Internet that you can use to actually record whatever happens on your desktop as well as your voice. The files are pretty big, though.
Also, if you've already downloaded the Smart Board software, you can use that to do the same thing. Again the files are pretty large.
You go to Smart Board Software and then you click on Smart Recorder.
Workshop!
question 1:

Everything in this question is pretty straight forward. Thanks to Graeme, its even colour coordinated. (:
Question 2:

The same applies for this question.
Question 3:

This is also pretty straight forward. You can put this into your calculator too.
Simply type in the equation into "Y=", then press "2nd" + "Calc". Press up so that you're at the bottom option and press enter. You'll be on the graph screen. Press "-1", "enter", "5", "enter". And you'll see the results. As a result, the answer is 12. Which Van has stated above. (:
That's all folks!
Next Scribe ...
Timmy!! .. (=
Bacchanalia: "Generally just a wine festival but really is a wine festival in honor of Dionysus. (Greek god of horses and drunkenness) (Poseidon is lord of horses)."
Del.icio.us
If you don't have a Del.icio.us account yet, you should make one!
Http://del.icio.us/
Your username should not include your last name. If you're having trouble you can add the class blog and your first name.
Example: Sandyapcal07
Assignment: Find one link for each unit that we've studied and add it to Del.icio.us. It can be anything, but at least put some effort into it and don't just pick the first website you see. So far you have two links to add, Pre Calculus and Derivatives.
Screen-O-Matic
This is a program on the Internet that you can use to actually record whatever happens on your desktop as well as your voice. The files are pretty big, though.
Also, if you've already downloaded the Smart Board software, you can use that to do the same thing. Again the files are pretty large.
You go to Smart Board Software and then you click on Smart Recorder.
Workshop!
question 1:

Everything in this question is pretty straight forward. Thanks to Graeme, its even colour coordinated. (:
Question 2:

The same applies for this question.
Question 3:

This is also pretty straight forward. You can put this into your calculator too.
Simply type in the equation into "Y=", then press "2nd" + "Calc". Press up so that you're at the bottom option and press enter. You'll be on the graph screen. Press "-1", "enter", "5", "enter". And you'll see the results. As a result, the answer is 12. Which Van has stated above. (:
That's all folks!
Next Scribe ...
Timmy!! .. (=
Friday, October 26, 2007
Thursday, October 25, 2007
Thursday's Post
The Word of the day brought to you by Graeme is Effervescence - a fizzy quality especially in wine
Today's class started off with a laugh. A kid had to find x in a question on a test,using his brain power and he circled x saying here it is.
After that it was back to business with the question: If a particle is moving in a straight line. Its position is given by s(t)= t squared- 3t +1. we had to find the change in position from t1 to t4.
simply plug in the values of t at both times (1,4) then subtract t1 from t4 to get your answer of 6.

We were then given a positive monotonic graph of a vehicle's speed per second. The time intervals are 2
Today's class started off with a laugh. A kid had to find x in a question on a test,using his brain power and he circled x saying here it is.
After that it was back to business with the question: If a particle is moving in a straight line. Its position is given by s(t)= t squared- 3t +1. we had to find the change in position from t1 to t4.
simply plug in the values of t at both times (1,4) then subtract t1 from t4 to get your answer of 6.

We were then given a positive monotonic graph of a vehicle's speed per second. The time intervals are 2
* note-- the quick way of find lower and higher estimates are if
lower- add up all the speeds except last and multiply it by 2 ( 2 because time intervals are 2)
upper- add up all the speeds except first then multiply it by 2
max error is upper estimate subtract lower
The fundamental theorem ( might be bad quality)

I finally found a way to put on a picture :) self pat on shoulder, I'm not much of a computer guy unless its playing games so its a accomplishment on my part

these are kinda fuzzy but it's asking whats the change in.
I believe is is important to know.
The last of the class was spent doing questions on smart board and practising different methods to get the answer. 

this is all, I think I got it all covered and Il try to improve my scribes in the future. The next scribe is Graeme---just because he cant beat me in magic :) if he can't do it then I will choose someone else, peace
Wednesday's Scribe
This will be Wednesday's post just coming a bit late, sorry. I'm new to this whole thing so if I miss anything let me know and I will edit anything I have to, just talk to me in class. Thanks.
As for Graeme's selected word for this day I lost it somewhere today and I will ask him for another if he has.
We started class as usual, can't remember anything special happening.
The teacher gave as an assignment of the smart board and we had to figure out the question with our new program.
[slideshare id=145852&doc=ap-calculus-slides-october-24-2007-1193346057870243-5&w=425]
* note--If the equation is f(x)= to a square root over (a number minus x squared)
then the graph will be a half Circle over the X axis.
* note--The trapezoid sum is A ={(B+b)h}/2
If that's not clear, sorry, but its supposed to be area of trapezoid = Big base+ small base multiplied by the interval then multiply all that by 1/2 .
After we learn about the Definite Integral and how to calculate that in many different ways on calculator,and how to calculate the number of intervals so that the estimate of the integral will be off by a set percentage of the actual integral.
*note--If you guys want let me know and I will make a more detailed information on definite integral,I dont know how detailed you guys like these posts to be.
that's all we did in class, seemed shorter then most for me. the next scribe will be me as I already missed so many scribe days so I want to make it for you guys :P so i hope this is okay if not let me know , peace
As for Graeme's selected word for this day I lost it somewhere today and I will ask him for another if he has.
We started class as usual, can't remember anything special happening.
The teacher gave as an assignment of the smart board and we had to figure out the question with our new program.
[slideshare id=145852&doc=ap-calculus-slides-october-24-2007-1193346057870243-5&w=425]
* note--If the equation is f(x)= to a square root over (a number minus x squared)
then the graph will be a half Circle over the X axis.
* note--The trapezoid sum is A ={(B+b)h}/2
If that's not clear, sorry, but its supposed to be area of trapezoid = Big base+ small base multiplied by the interval then multiply all that by 1/2 .
After we learn about the Definite Integral and how to calculate that in many different ways on calculator,and how to calculate the number of intervals so that the estimate of the integral will be off by a set percentage of the actual integral.
*note--If you guys want let me know and I will make a more detailed information on definite integral,I dont know how detailed you guys like these posts to be.
that's all we did in class, seemed shorter then most for me. the next scribe will be me as I already missed so many scribe days so I want to make it for you guys :P so i hope this is okay if not let me know , peace
Wednesday, October 24, 2007
Tuesday Scribe
Alright, I'm not supposed to be the scribe today, or maybe I was, but I wasn't sure. So, I'm doing it anyways, and doing it now, because I couldn't access my computer last night for a long duration of time. Anyways, I'll start with:
Graeme's Selected Word Of the Day: Foment
Stir up or worsen trouble.
Don't forget to double click the word Foment, if you want a more in depth definition. (That awesome tool at the side there)
The start of class begins with an online site that teaches calculus. In the middle of the lesson, we learn a new word.
Monotonic: The graph is strictly increasing or decreasing
(don't forget that awesome double click)
And slightly after that new word, Mr. K tells us about Riemann. And his life story. It's got love, death, but, a rather sad ending. When one day Mr. K tells us the story he REALLY thinks is it, then I'll post it up. But, during the time of story time, he thought he was mixing up his stories.
[Insert Riemann's story here]
But, really, when the smart board is there, it's a lot harder to do a scribe than when I used to do them. Because, all the pictures I'd draw are already on the slide show.
So, the online lesson continues... until the school network goes slow. Mr. K gets a little ticked off, and we start doing questions.
We take left hand sums and right hand sums, using n as the number of rectangles we break the curving graph into.
Left hand sums would be taking one of the extreme estimates.
Right hand sums would be taking the other extreme estimate.
(I think) Depending on the graph, if it's Monotonic Increasing or Decreasing, taking left and right hand sums, wouldn't be the same on both graphs, because you're measuring for different extreme estimates. (I don't even know if that made sense)
And our class ended there.
And a side note. If you're ever one of those people that type "lol" all the time, it's to indicate that it's comical, and probably also saying "okay, your turn to say something".
Homework for... uhh... tonight...yes. Tonight(last night) is: Exercise 3.2 All the odds.
Next scribe is... Craig.
lol
Graeme's Selected Word Of the Day: Foment
Stir up or worsen trouble.
Don't forget to double click the word Foment, if you want a more in depth definition. (That awesome tool at the side there)
The start of class begins with an online site that teaches calculus. In the middle of the lesson, we learn a new word.
Monotonic: The graph is strictly increasing or decreasing
(don't forget that awesome double click)
And slightly after that new word, Mr. K tells us about Riemann. And his life story. It's got love, death, but, a rather sad ending. When one day Mr. K tells us the story he REALLY thinks is it, then I'll post it up. But, during the time of story time, he thought he was mixing up his stories.
[Insert Riemann's story here]
But, really, when the smart board is there, it's a lot harder to do a scribe than when I used to do them. Because, all the pictures I'd draw are already on the slide show.
So, the online lesson continues... until the school network goes slow. Mr. K gets a little ticked off, and we start doing questions.
We take left hand sums and right hand sums, using n as the number of rectangles we break the curving graph into.
Left hand sums would be taking one of the extreme estimates.
Right hand sums would be taking the other extreme estimate.
(I think) Depending on the graph, if it's Monotonic Increasing or Decreasing, taking left and right hand sums, wouldn't be the same on both graphs, because you're measuring for different extreme estimates. (I don't even know if that made sense)
And our class ended there.
And a side note. If you're ever one of those people that type "lol" all the time, it's to indicate that it's comical, and probably also saying "okay, your turn to say something".
Homework for... uhh... tonight...yes. Tonight(last night) is: Exercise 3.2 All the odds.
Next scribe is... Craig.
lol
Tuesday, October 23, 2007
Monday, October 22, 2007
Scribe Post # 3
Hello again, I am today's scribe. Today was the first lesson of the unit "The Definite Integral". So there was not a lot of information that was covered in class today except the basics of the unit The Definite Integral. If you take a look at the second slide that Mr. K posted just below this scribe post you will see a question like; Give a lower and upper estimate of the distance traveled in 5 seconds.
In order to find the lower estimated distance you must add all the values on the table excluding the last value of velocity at 5 seconds which is 5.7 ft/s. The reason why you must exclude the 5.7 ft/s is because the lower estimate should not have the highest velocity which was recorded. As even with the high estimated distance you do not count the lowest value on the table, because you want to find the highest estimated distance, in this case 1.4 ft/s is not used. So the lower estimated value is 1.4 + 2.7 + 3.5 + 4.5 + 5 = 17.1 the reason why we are adding is because the values in change of time is 1 so 1.4 divided by 1 is still 1.4. The upper estimated value is 2.7 + 3.5 + 4.5 + 5.0 + 5.7 = 21.4.
If you move to the third slide it asks for the actual distance travel. Well you are never going to find the actual distance, only the approximate distance travel. So you begin by adding the upper and lower estimated values and divide by two ( like when trying to get the average of two numbers). Now in this case the value is 19.25 Although there must be an error because more than likely the actual distance is not the average. So we say that there is an error of + or - 2.15.
As there is a distance of 2.15 from 19.25 to either 17.1 or 21.4.
If you move to the fourth slide it asks for representation of the lower estimate, well it is the blue graph, as it is lower then the red graph, which means it is the lower estimate.
If you move to the fifth graph it asks to fill out the rest of the table and pick values for the 0.5 second interval ( which cannot be the average of the whole seconds) so intern once done picking values find the lower and upper estimates in my case I had gotten lower: 18.25 upper: 20.4 . (When finding the estimates you have to divide by 2 because of the 0.5 second intervals) Then find the actual distance in this case after adding and dividing by 2 and you received 19.325. In this case also we received the same error which is + or - 1.075. So in other words the shorter the interval of time, the smaller the error for actual distance.
So that was the class, not much information, but a lot of knowledge on Definite Integrals.
Next class scribe will be Mark.
In order to find the lower estimated distance you must add all the values on the table excluding the last value of velocity at 5 seconds which is 5.7 ft/s. The reason why you must exclude the 5.7 ft/s is because the lower estimate should not have the highest velocity which was recorded. As even with the high estimated distance you do not count the lowest value on the table, because you want to find the highest estimated distance, in this case 1.4 ft/s is not used. So the lower estimated value is 1.4 + 2.7 + 3.5 + 4.5 + 5 = 17.1 the reason why we are adding is because the values in change of time is 1 so 1.4 divided by 1 is still 1.4. The upper estimated value is 2.7 + 3.5 + 4.5 + 5.0 + 5.7 = 21.4.
If you move to the third slide it asks for the actual distance travel. Well you are never going to find the actual distance, only the approximate distance travel. So you begin by adding the upper and lower estimated values and divide by two ( like when trying to get the average of two numbers). Now in this case the value is 19.25 Although there must be an error because more than likely the actual distance is not the average. So we say that there is an error of + or - 2.15.
As there is a distance of 2.15 from 19.25 to either 17.1 or 21.4.
If you move to the fourth slide it asks for representation of the lower estimate, well it is the blue graph, as it is lower then the red graph, which means it is the lower estimate.
If you move to the fifth graph it asks to fill out the rest of the table and pick values for the 0.5 second interval ( which cannot be the average of the whole seconds) so intern once done picking values find the lower and upper estimates in my case I had gotten lower: 18.25 upper: 20.4 . (When finding the estimates you have to divide by 2 because of the 0.5 second intervals) Then find the actual distance in this case after adding and dividing by 2 and you received 19.325. In this case also we received the same error which is + or - 1.075. So in other words the shorter the interval of time, the smaller the error for actual distance.
So that was the class, not much information, but a lot of knowledge on Definite Integrals.
Next class scribe will be Mark.
Friday, October 19, 2007
BoB 1
Well, this unit was a unit that I had struggled with. It was all alright until the day Mr.K introduced the graphing of a function to find its derivative and second derivative. After doing all the homework and discussing it with other students in the class I felt more reassured, although many times I do still get confused on the second derivative of the parent function. All in all i believed i had studied enough and get the understanding of derivative functions. So good luck to everyone on today's derivative test.
BOB
So here we are bobbing before another test. This unit has been somewhat difficult for me. However with my lovely boyfriend, Manny, I can usually clear things up quite well. If i concentrated a tad bit more i think things would be a lot easier right now. I can always change for the next units. (: It's hard to devote all your time to school when there's so many other things going on. Committees and so on and so forth. I know i should try a little harder next time though..
anyway. Good luck to everyone on the test today!
anyway. Good luck to everyone on the test today!
Thursday, October 18, 2007
Scribulous
Alright a pretest scribe! I guess I have some explaining to do.... about the questions on the pretest!
Question 1)
If f(x) = ln(sqr(x)) then the average rate of change on the interval [3,7] is... then 5 possible answers. Using Roberts method you punch the equation into the calculator and use that handy-dandy slope program and out pops the answer or you can do it manually by hand and come up with A) 0.106
Question 2)
Suppose that the number of bacteria in a certain organism grows over time and the number, N(t), of bacteria (measured in thousands) at any time is given by:
N(t) = t(2 + cos(t))^(4/3) + 3t
At approximately what time ,t, in the first ten days is the colony growing the fastest?
First and foremost I must remind you all that IN THIS CLASS CALCULATORS SHOULD BE IN RADIANS! It is truly *very* annoying to miss a mark because of your calculator...
Now entered into your calculator you turn on that oh so useful derivative-of-whatever-you-have-in-your-Y1-slot graph and you look for the peak! (Then use that maximum function to give you a value)
The answer is.... 5.18!
Question 3)
Limit as x approaches one of:
(2x^2 + x - 3)/(3x^2 -x -2)
is equal to.... ?.
Well first you try putting one in right away and find your dividing by 0!
Algebraic massage required...
These both factor readily and two of the terms reduce. Then 1 can be entered in and you end up with 5/5 or 1... which is the answer!
Question 4)
The graph of the second derivative of a function f is shown at the right. Which of the following statements are true (I realize that there is no graph there but there but there is on this slide)
I) The graph of f has an inflection point at x = -1.
II) The f graph is concave down on the interval (-1,3).
III) The graph of the derivative function f prime is increasing at x = 1.
Then you find which of those statements is true.
I) An inflection point on a second derivative graph would be a zero and there happens to be one where they say that there is an inflection point therefore it is true!
II) The second derivative measures concavity, since it is negative on the interval that they say then the statement is true!
III) The second derivative is a measure of the slope of the first derivative. Since the value is negative the slope of that point is negative so it is decreasing at that point making this statement false.
So the answer is I and II are true!
Question 5)
Suppose a function f is defined so that is has derivatives:
f'(x) = x^2(x-1) and f''(x) = x(3x-2)
Over what intervals is f both increasing and concave up?
Well for it to be increasing the first derivative must be positive. Finding the roots of that we find them to be x = 0 and 1. Then do a sign check by entering a value before, in between and after to find when it is positive. The process is repeated for the second derivative because where that is positive the graph is concave up. We find the overlapping positive areas to be when x > 1!
Question 6)
a) Condider the following table of data.
Estimate f'(5.2) as accurately as possible.
Well f' would be the slope at that point!
Since they are on equal intervals and there are values before and after 5.2 we are able to use the symmetric difference quotient to get a more accurate result. It is found by finding the slope of that point with the points before and after and averaging it which results in you getting -9/4.
b) Write and equation for the slope of the tangeant line at that point.
Well... you are given a point.... you are given a slope... so let's use point slope form shall we? and you end up with y - 8.8 = -2.25 (x - 5.2)
c) Use your answer in part (b) to approximate f'(5.26)
Since the two points are so close together we can just plug that value into the equation we made to get a fairy accurate answer... which ends up being 8.665.
d) What is the sign of f''(5.2)? Explain.
Well this one I got wrong. I drew a graph where this point really looked like a point of inflection and that's what I based my answer on. WRONG! Mr.K ends up saying something along the lines of "Using the algebra to prove a point is far better then the graphical approach and will be expected of you on the exam". Since f' is decreasing to the left and right of this point we can deduce that this point is also decreasing meaning the graph is concave down meaning that f'' is negative at that point! (Though I still think it could be a point of inflection, but I do see the logic in what was said).
Woo that was a workout for the fingers!
The next scribe will be DINO!
Question 1)
If f(x) = ln(sqr(x)) then the average rate of change on the interval [3,7] is... then 5 possible answers. Using Roberts method you punch the equation into the calculator and use that handy-dandy slope program and out pops the answer or you can do it manually by hand and come up with A) 0.106
Question 2)
Suppose that the number of bacteria in a certain organism grows over time and the number, N(t), of bacteria (measured in thousands) at any time is given by:
N(t) = t(2 + cos(t))^(4/3) + 3t
At approximately what time ,t, in the first ten days is the colony growing the fastest?
First and foremost I must remind you all that IN THIS CLASS CALCULATORS SHOULD BE IN RADIANS! It is truly *very* annoying to miss a mark because of your calculator...
Now entered into your calculator you turn on that oh so useful derivative-of-whatever-you-have-in-your-Y1-slot graph and you look for the peak! (Then use that maximum function to give you a value)
The answer is.... 5.18!
Question 3)
Limit as x approaches one of:
(2x^2 + x - 3)/(3x^2 -x -2)
is equal to.... ?.
Well first you try putting one in right away and find your dividing by 0!
Algebraic massage required...
These both factor readily and two of the terms reduce. Then 1 can be entered in and you end up with 5/5 or 1... which is the answer!
Question 4)
The graph of the second derivative of a function f is shown at the right. Which of the following statements are true (I realize that there is no graph there but there but there is on this slide)
I) The graph of f has an inflection point at x = -1.
II) The f graph is concave down on the interval (-1,3).
III) The graph of the derivative function f prime is increasing at x = 1.
Then you find which of those statements is true.
I) An inflection point on a second derivative graph would be a zero and there happens to be one where they say that there is an inflection point therefore it is true!
II) The second derivative measures concavity, since it is negative on the interval that they say then the statement is true!
III) The second derivative is a measure of the slope of the first derivative. Since the value is negative the slope of that point is negative so it is decreasing at that point making this statement false.
So the answer is I and II are true!
Question 5)
Suppose a function f is defined so that is has derivatives:
f'(x) = x^2(x-1) and f''(x) = x(3x-2)
Over what intervals is f both increasing and concave up?
Well for it to be increasing the first derivative must be positive. Finding the roots of that we find them to be x = 0 and 1. Then do a sign check by entering a value before, in between and after to find when it is positive. The process is repeated for the second derivative because where that is positive the graph is concave up. We find the overlapping positive areas to be when x > 1!
Question 6)
a) Condider the following table of data.
Estimate f'(5.2) as accurately as possible.
Well f' would be the slope at that point!
Since they are on equal intervals and there are values before and after 5.2 we are able to use the symmetric difference quotient to get a more accurate result. It is found by finding the slope of that point with the points before and after and averaging it which results in you getting -9/4.
b) Write and equation for the slope of the tangeant line at that point.
Well... you are given a point.... you are given a slope... so let's use point slope form shall we? and you end up with y - 8.8 = -2.25 (x - 5.2)
c) Use your answer in part (b) to approximate f'(5.26)
Since the two points are so close together we can just plug that value into the equation we made to get a fairy accurate answer... which ends up being 8.665.
d) What is the sign of f''(5.2)? Explain.
Well this one I got wrong. I drew a graph where this point really looked like a point of inflection and that's what I based my answer on. WRONG! Mr.K ends up saying something along the lines of "Using the algebra to prove a point is far better then the graphical approach and will be expected of you on the exam". Since f' is decreasing to the left and right of this point we can deduce that this point is also decreasing meaning the graph is concave down meaning that f'' is negative at that point! (Though I still think it could be a point of inflection, but I do see the logic in what was said).
Woo that was a workout for the fingers!
The next scribe will be DINO!
BOB and the chocolate factory
My first bob for this class and for the year. I think that the overall course hasn't presented a lot of trouble, though I feel like a broken BOB record after saying that too frequently. I have encountered much of this unit prior to the course, so quite a bit of it is a review for me, though there were some questions that required some serious concentration.
These questions put my brain to work, but the more practice I embarked upon regarding these types of questions they inevitably sparked a further comprehension of the overall unit and of all of the unit's constituent areas. I cannot stress the significance of the excercises enough. Well, as for Mr. K, I'm sure he will optimize these questions and utilize them to the maximum extent possible to challenge us on tomorrow's test. But the test itself should not be too difficult, just don't forget to convert your calculator mode from DEGREES to RADIANS, just in case some trig questions present themselves similar to counteract the consequences of today's fiasco (sorry to hear your calc cost you that mark Graeme).
I'll probably just end this bob with a couple quick notes for the class:
When f has a max or a min at x=a, f' will have a root at x=a.
When f has an point of inflection (change in concavity) at x=a, then f'' will have a root at x=a.
When f is increasing, f' is positive. When f is decreasing, f' is negative.
When f is concave up, f'' is positive. When f is concave down, f'' is negative.
When f' has a max or a min at x=a, f'' will have a root at x=a.
Well that concludes my BOB post for today, I tried to keep it as short as possible, I'd just like to wish everyone luck on tomorrow's test and I want to remind everyone to study!
GOOD LUCK! and GOOD NIGHT!
These questions put my brain to work, but the more practice I embarked upon regarding these types of questions they inevitably sparked a further comprehension of the overall unit and of all of the unit's constituent areas. I cannot stress the significance of the excercises enough. Well, as for Mr. K, I'm sure he will optimize these questions and utilize them to the maximum extent possible to challenge us on tomorrow's test. But the test itself should not be too difficult, just don't forget to convert your calculator mode from DEGREES to RADIANS, just in case some trig questions present themselves similar to counteract the consequences of today's fiasco (sorry to hear your calc cost you that mark Graeme).
I'll probably just end this bob with a couple quick notes for the class:
When f has a max or a min at x=a, f' will have a root at x=a.
When f has an point of inflection (change in concavity) at x=a, then f'' will have a root at x=a.
When f is increasing, f' is positive. When f is decreasing, f' is negative.
When f is concave up, f'' is positive. When f is concave down, f'' is negative.
When f' has a max or a min at x=a, f'' will have a root at x=a.
Well that concludes my BOB post for today, I tried to keep it as short as possible, I'd just like to wish everyone luck on tomorrow's test and I want to remind everyone to study!
GOOD LUCK! and GOOD NIGHT!
BOB
This unit about derivatives was really tough for me. It started off relatively easily until the concepts just kept coming and coming, but as the days go by and with the continued help from my peers, i am starting to understand the concepts a little bit more. I just hope that my knowledge will be sufficient for the test tomorrow. Well, thats it. Good luck to everyone and study hard!
BOB
Hello everyone! The time has come again to bob. Well this unit was a tough one for me and I found it really challenging. With so many concepts to soak in, I really felt overwhelmed with this unit. I'm starting to understand more and more each day and hope to know enough for the test. Well good luck to everyone on the test and best wishes. Bye for now and don't forget to study!
Wednesday, October 17, 2007
ßØß
Well my first bob of the year... I must say I have been finding the base concepts of this unit fairly easy. But my problem is with the recognition of those base concepts. They are as straight forward as can be in the text but I know that Mr.K is never that straight forward. I just have to work on identification of what is given in the question and use the knowledge that I'm comfortable with to get the questions. If I can do that this test will be a cake walk.
Gute Nacht!
GreyM
Gute Nacht!
GreyM
Blogging on Blogging..
Hey! It's Tim-Math-Y and its time to b.o.b. again. Well this new unit was definitive.. different, as it included a new idea, Derivatives. At first this unit really had my mind twisting up on itself trying to visualize a derivative of a function, then holding that image, attempting to visualize the second derivative. I will admit two things. That wasn't a good idea to imagine similtaneously and second, that I'm still a little confused about the matter.
However, thinking back of the leaked characteristics of the power law, which I also admit that I don't know how it works.. yet, it helps me remember that each derivative is a degree down from its parent (cubic>quadratic>linear etc.), and thus aids me in picturing the graphs.
Now with the knowledge of derivatives, it brings fourth questions that we deal with in physics, such as comparing two variables: time and distance, time and velocity, time and acceleration etc. I find that I don't have many problems with these types of Qs because I understand the concepts to a more complete level.
So I can say that I'm semi-prepared for derivative functions and their like. Other than that, I still don't really understand the exact purpose and use of finding limits. Limits still greatly puzzles me as, logically, it just isn't registering for me. Besides that, Continuity, I understand and I'm glad I can say that atleast...
Wow, my bob is long? =\ Good luck on the test on friday guys!
However, thinking back of the leaked characteristics of the power law, which I also admit that I don't know how it works.. yet, it helps me remember that each derivative is a degree down from its parent (cubic>quadratic>linear etc.), and thus aids me in picturing the graphs.
Now with the knowledge of derivatives, it brings fourth questions that we deal with in physics, such as comparing two variables: time and distance, time and velocity, time and acceleration etc. I find that I don't have many problems with these types of Qs because I understand the concepts to a more complete level.
So I can say that I'm semi-prepared for derivative functions and their like. Other than that, I still don't really understand the exact purpose and use of finding limits. Limits still greatly puzzles me as, logically, it just isn't registering for me. Besides that, Continuity, I understand and I'm glad I can say that atleast...
Wow, my bob is long? =\ Good luck on the test on friday guys!
Calculus BOB saluclaC
Well, BOBin' for the first time in Calculus... haha we got away with one last week.
Hmmm... well for anyone who thought Calculus should have been a little harder while doing the first unit, HERE YOU GO! Holy, honnestly, I did not expect it to be this big of a jump from the most familiar things to a brand new concept of Derivatives and Limits. WOW!!! Anyway, now I think I'm finally starting to get going with this stuff in the sense that I know EXACTLY WHAT I'M DOING!!! At the beginning of the unit, with two different teachers teaching, I found it a little difficult to stay on track. However, lately I've started to understand it a little more. One thing that I know I will probably have a little difficulty with is the limits portion... I never really knew exactly what was happening with them. Oh well, I'll see how they are in the review tonight. Other than that I think I should be set, although I'm sure I'll make a couple mistakes... nobody's perfect (cough*Chris*cough) LOL!!! just kidding....
Oh! Almost forgot! REMEMBER:
Hmmm... well for anyone who thought Calculus should have been a little harder while doing the first unit, HERE YOU GO! Holy, honnestly, I did not expect it to be this big of a jump from the most familiar things to a brand new concept of Derivatives and Limits. WOW!!! Anyway, now I think I'm finally starting to get going with this stuff in the sense that I know EXACTLY WHAT I'M DOING!!! At the beginning of the unit, with two different teachers teaching, I found it a little difficult to stay on track. However, lately I've started to understand it a little more. One thing that I know I will probably have a little difficulty with is the limits portion... I never really knew exactly what was happening with them. Oh well, I'll see how they are in the review tonight. Other than that I think I should be set, although I'm sure I'll make a couple mistakes... nobody's perfect (cough*Chris*cough) LOL!!! just kidding....
Oh! Almost forgot! REMEMBER:
13-0-13
Well...it is time for me to bob again. It's the first of my last this year. Well it seems to me that my muddiest point was the whole unit. It has really confused me a lot. I always had to get someone else to explain things to me. (Thanks Chris, Graeme, Craig, Robert) haha. I understand that a derivative is the slope of the rate of change. I get how first and second derivatives work. Continuity kind of confuses me. I think it's when I read a question that I get all mixed up because I don't quite understand it but if I read it over again and try to understand what it's asking me then I will be able to get it. In class I seem to get things more than when I'm just doing a question by myself...it's weird how that works and I think I'm not the only one that happens to. I really like the feeling of when I understand something, it's like yay! lightbulb! That only happens sometimes though. Overall, I found this unit quite challenging. I will try my best to decipher what is in front of me and try to solve it carefully.
Good luck to everyone on the test and pretest! =)
Good luck to everyone on the test and pretest! =)
The Scribe that didn't know he was the scribe until 3:33...
Haha, hmmm.... well to make a long story short, I looked at the blog briefly last night because I thought there was homework that needed to be posted (there wasn't) and while I was there, I completely forgot to check who the next scribe was. (evidently it was me). I learned from Aichelle a couple minutes after the end of class that I was, fortunately the class wasn't very complicated and no new concpets were really introduced. So, here we go with probably the easiest scribe post I've had to do in a while.
Well, this class was a "workshop" class, so we got into our groups and took a look at the first question which seemed very familiar. It has to do with the graph of the height of a thrown ball over time (Grade 11 Pre-Cal question). However he added the velocity of the ball which is the rate of change (a.k.a. derivative) of the function. Then we were asked a series of questions about the scenario. For question and answer, click the "first question link".
Question Two basically asked us to find the slopes of the tangent at each point and list them in increasing order.
The third question was a little interesting because it gave us a told us that an original function was transformed by with a vertical shift. Then it asked us about how the derivative of the transformed function related to that of the original function. Well, when a function is shifted vertically, the vertical and horizontal scale remains the same (same size), as does the shape and it's position along the x-axis (x coordinates). The only thing that differs between it and it's original function is the y coordinates. Now, we know that a derivative is a graph of the slope of the tangents of the parent function vs. the points along the x-axis. So, since the shape, size, and x coordinates of the transformed function do not change, the slope of tangent at each point remain the same meaning the derivatives are the same!!!.
When Mr. K. clicked the next slide button on the SmartBoard Question Four came on the screen. This dealt with the derivatives of even and odd functions. Basically, the derivative of a point [ie. (4,2)] on a even function will result in a derivative that is equal, but opposite at the point that is opposite about the y-axis [(-4,2)]. When dealing with an odd function, the derivative at one point [ie. (1,3)] will be the exact same as the derivative of the point opposite about the origin [(-4,-2)].
Finally, we finished with two questions that were connected. Question Five was the last one we worked with, but has the same rule as Question Six which is to be completed and posted in the comments box this evening. For an explanation of how to do these two, click here.
Well, that concludes my scribe post for the night. I shall post my answer to the Homework question in the comment box now that at least some people have tried to answer it.
Don't Forget:
-PRETEST TOMORROW!!!
-TEST ON FRIDAY!!!
-B.O.B. BEFORE THE TEST (excluding Aichelle as she has already done so)
LAST BUT NOT LEAST, THE NEXT SCRIBE WILL BEEEEEEE:
...
...
...
once again I pick GREY M!!!
good night =D
Well, this class was a "workshop" class, so we got into our groups and took a look at the first question which seemed very familiar. It has to do with the graph of the height of a thrown ball over time (Grade 11 Pre-Cal question). However he added the velocity of the ball which is the rate of change (a.k.a. derivative) of the function. Then we were asked a series of questions about the scenario. For question and answer, click the "first question link".
Question Two basically asked us to find the slopes of the tangent at each point and list them in increasing order.
The third question was a little interesting because it gave us a told us that an original function was transformed by with a vertical shift. Then it asked us about how the derivative of the transformed function related to that of the original function. Well, when a function is shifted vertically, the vertical and horizontal scale remains the same (same size), as does the shape and it's position along the x-axis (x coordinates). The only thing that differs between it and it's original function is the y coordinates. Now, we know that a derivative is a graph of the slope of the tangents of the parent function vs. the points along the x-axis. So, since the shape, size, and x coordinates of the transformed function do not change, the slope of tangent at each point remain the same meaning the derivatives are the same!!!.
When Mr. K. clicked the next slide button on the SmartBoard Question Four came on the screen. This dealt with the derivatives of even and odd functions. Basically, the derivative of a point [ie. (4,2)] on a even function will result in a derivative that is equal, but opposite at the point that is opposite about the y-axis [(-4,2)]. When dealing with an odd function, the derivative at one point [ie. (1,3)] will be the exact same as the derivative of the point opposite about the origin [(-4,-2)].
Finally, we finished with two questions that were connected. Question Five was the last one we worked with, but has the same rule as Question Six which is to be completed and posted in the comments box this evening. For an explanation of how to do these two, click here.
Well, that concludes my scribe post for the night. I shall post my answer to the Homework question in the comment box now that at least some people have tried to answer it.
Don't Forget:
-PRETEST TOMORROW!!!
-TEST ON FRIDAY!!!
-B.O.B. BEFORE THE TEST (excluding Aichelle as she has already done so)
LAST BUT NOT LEAST, THE NEXT SCRIBE WILL BEEEEEEE:
...
...
...
once again I pick GREY M!!!
good night =D
Tuesday, October 16, 2007
Blogging On Blogging AKA BOB
We were talking about exactly what sort of post you're supposed to make to get that one blogging mark on your test. The kind of post I'd like you to make should have one or more of these characteristics:
Your posts do not have to be long. I'm far more interested in the quality of what you write rather than the quantity.
Make certain you always use 3 labels on your post: [your name], [unit tag], BOB
When you share where you are in your learning a few days before the unit test I can address those issues in class so, hopefully, you will get much more than one extra mark on the test. ;-)
Happy Blogging!
- Write about what you understand the least in the unit so far; your personal "Muddiest Point."
- A reflection on a particular class.
- A reflective comment on your progress in the course.
- A comment on something that you've learned that you thought was "cool".
- A comment about something that you found very hard to understand but now you get it! Describe what sparked that "moment of clarity" and what it felt like.
- Have you come across something we discussed in class out there in the "real world" or another class? Describe the connection you made.
Your posts do not have to be long. I'm far more interested in the quality of what you write rather than the quantity.
Make certain you always use 3 labels on your post: [your name], [unit tag], BOB
When you share where you are in your learning a few days before the unit test I can address those issues in class so, hopefully, you will get much more than one extra mark on the test. ;-)
Happy Blogging!
Scribe version 3: Continuing our discussion of continuity
Well, we started off today's class by forming different groups and working on two problem solving questions about derivatives and continuity.
For the first part of the first question G`(t) represents the exact definition of a derivative so in this case we can see that G(x+h)=log((9+Δx)+1). Therefore G(x)=log (x+1). It can be seen on the second slide.
For the second part of the question since x=9 ,therefore a=9 just by looking at how g`(t) represent the exact definition of a derivative.
Now, for the second question it will be a nonremovable discontinuity. WHY? It is a nonremovable discontinuity because a limit has to exist in order for this to be removable. It can be seen on the third slide.
For the second part of the question the answer would have to be NO, because if you buy more than 3 bags of grain from this company the price jumps up. As it can be seen on the graph of the piece wise function that after exceeding three bags of grain the behavior of the function changes for being linear into being quadratic.
Then after doing those two questions we spent the rest of our time, taking a quiz at visual calculus.
Thats it for today. Our homework for tonight is to start doing the supplementary questions for chapter 2. The next scribe will be......(drum rolls)...CRAIG. (sorry but i have to pick someone)
For the first part of the first question G`(t) represents the exact definition of a derivative so in this case we can see that G(x+h)=log((9+Δx)+1). Therefore G(x)=log (x+1). It can be seen on the second slide.
For the second part of the question since x=9 ,therefore a=9 just by looking at how g`(t) represent the exact definition of a derivative.
Now, for the second question it will be a nonremovable discontinuity. WHY? It is a nonremovable discontinuity because a limit has to exist in order for this to be removable. It can be seen on the third slide.
For the second part of the question the answer would have to be NO, because if you buy more than 3 bags of grain from this company the price jumps up. As it can be seen on the graph of the piece wise function that after exceeding three bags of grain the behavior of the function changes for being linear into being quadratic.
Then after doing those two questions we spent the rest of our time, taking a quiz at visual calculus.
Thats it for today. Our homework for tonight is to start doing the supplementary questions for chapter 2. The next scribe will be......(drum rolls)...CRAIG. (sorry but i have to pick someone)
Monday, October 15, 2007
Derivative Assignment
Well here is my derivative assignment finally being posted up on the blog.  For the answers to my derivative question, I will create a comment on this very post detailing the answers for my derivative question.
For the answers to my derivative question, I will create a comment on this very post detailing the answers for my derivative question.
 For the answers to my derivative question, I will create a comment on this very post detailing the answers for my derivative question.
For the answers to my derivative question, I will create a comment on this very post detailing the answers for my derivative question.
Scribe post # 3
[filling in for Mark, he will be tomorrow's scribe]
In today's class we talked about the assignment we had on Friday, which was to create a problem identifying which 3 out of the 4 functions were related. Next, we talked about the hits we are getting from around the world. The map can be seen on the right, if you scroll down. After that, we searched for a wonderful picture to start of the slides with. It's the picture with the swirly smarties! Yay! Smarties = Yummy!
Mr. K. then brought up the term continuous functions. He asked us what we thought it meant. After a short discussion (and spelling analysis) about continuous functions we came up with an informal definition. We said that a continuous function is a function that we can draw without lifting the pencil off the paper at any point. If you cannot draw the graph without lifting the pencil then it is not continuous. We also concluded that all polynomial functions are continuous functions (X², X³...). Functions like 1/X are not continuous. Absolute value functions can be continuous depending on the kind of function. When a function contains a hole/corner/cusp it is not a continuous function. If a function is differentiable then it is continuous. However, if the function is continuous it does not mean it is differentiable.
We also learned how to Factor a Difference of Cubes and Factor By Grouping.
***Factoring By Grouping should be learned in grade ten, although it is not in the curriculum. Since a number of us didn't learn it, we were taught/re-taught it today.
See slide 10.

On slide eleven we talked about the Intermediate Value Theorem and Corollary. A corollary means it does not need to be proven; follows naturally. On slide twelve, we talked about the Extreme Value Theorem. We said that if a function has a solution it has a root and if it is continuous it has to have a min and a max aka extreme values.
Homework: 2.8 ODDS up to 13 + 2
Tomorrow's scribe will be Mark.
***sorry if I did anything wrong...please tell me if I did so I can fix it.
In today's class we talked about the assignment we had on Friday, which was to create a problem identifying which 3 out of the 4 functions were related. Next, we talked about the hits we are getting from around the world. The map can be seen on the right, if you scroll down. After that, we searched for a wonderful picture to start of the slides with. It's the picture with the swirly smarties! Yay! Smarties = Yummy!
Mr. K. then brought up the term continuous functions. He asked us what we thought it meant. After a short discussion (and spelling analysis) about continuous functions we came up with an informal definition. We said that a continuous function is a function that we can draw without lifting the pencil off the paper at any point. If you cannot draw the graph without lifting the pencil then it is not continuous. We also concluded that all polynomial functions are continuous functions (X², X³...). Functions like 1/X are not continuous. Absolute value functions can be continuous depending on the kind of function. When a function contains a hole/corner/cusp it is not a continuous function. If a function is differentiable then it is continuous. However, if the function is continuous it does not mean it is differentiable.
We also learned how to Factor a Difference of Cubes and Factor By Grouping.
***Factoring By Grouping should be learned in grade ten, although it is not in the curriculum. Since a number of us didn't learn it, we were taught/re-taught it today.
See slide 10.

On slide eleven we talked about the Intermediate Value Theorem and Corollary. A corollary means it does not need to be proven; follows naturally. On slide twelve, we talked about the Extreme Value Theorem. We said that if a function has a solution it has a root and if it is continuous it has to have a min and a max aka extreme values.
Homework: 2.8 ODDS up to 13 + 2
Tomorrow's scribe will be Mark.
***sorry if I did anything wrong...please tell me if I did so I can fix it.
Sunday, October 14, 2007
Derivative assignment
assignment
Okay here is my post for the assignment..I hope I did it right and it shows up okay...=)
Labels:
Aichelle,
assignment,
Derivative Functions,
Homework
Graphs, graphs, graphs.....
I don't know why the text are really small after i convert the notebook files on pdf but please just bare with it and enjoy.^__^
Saturday, October 13, 2007
Scribe Post 2
Well the class was suppose to start with a quiz, but due to a part of the class not being there at the time of the quiz it was postponed until Monday. So instead we began class by programming a program into our calculators called intercept. It is on page 122 in the calculus text book that Mr. K gave out to all of us if any one has still not programmed their calculators. After programming the intercept program, Mr.K split us into groups where we did 2 problems which are just below this post on Mr.K class slides. That was basically Thursday's class, and for homework Mr.K wants us to create a problem like that on the first slide that Mr.K posted. Make four functions on one graph in which three functions are related by f(x), f'(x), and f"(x). and the fourth function to be unrelated from the other 3 functions and post it to the blog. The next scribe will be Mark.
Thursday, October 11, 2007
Today's Slides: October 11
Here they are ...
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
Labels:
Derivative Functions,
Mr. Kuropatwa,
Slides
Wednesday Scribe
Okay, it's me doing the scribe, again. Woohoo. I should do it for the 3rd time. Just to set some odd record. But, then again, it wouldn't be much of an entire class contributing, and more just like 1 person doing more. Anyways.
And btw, I did start BoB (blogging on blogging). I was the first to call it that, on my very first BoB. So there. You can even go WAY back and check it. (if you're really that bored)
So, Wednesday class. We began with just refreshing information about yesterday's work, with the f' and f''. Next up was looking at the worksheet that was given to us yesterday. Given f' how is Willy moving and does Roo beat Willy. Roo beats Willy, because Roo travels constantly, backwards. But, Willy does too, except he goes forward for a while. Then backwards. Rather odd assignment, but it generated a few good laughs and what not.
We then started looking at limits and concepts. The Sum, Difference, Product, and Quotient between 2 different Limits. The rules are simple. You can get some examples from the site Mr. K is going to put in the side bar. Simply Calculus I think it was called?
And for homework, we have section 2.8 all odd, and 24, and 30.
Horray. My job is finished... I guess. Sorry for the shortness and cheapness that it may appear in. But, for the most part, if I ever needed pictures, it's all in Mr. K's slides. Except for the ones that Mrs. (forgot her name) drew on the board. Which I need to add into my Tuesday scribe. Soon...
Thursday scribe. Whoever doesn't mind. I'll pick in class. Seeing the reaction should be worth it. Cya all in... about 6 hours. It's 8am when I made this scribe and yesterday's. Haha.
And btw, I did start BoB (blogging on blogging). I was the first to call it that, on my very first BoB. So there. You can even go WAY back and check it. (if you're really that bored)
So, Wednesday class. We began with just refreshing information about yesterday's work, with the f' and f''. Next up was looking at the worksheet that was given to us yesterday. Given f' how is Willy moving and does Roo beat Willy. Roo beats Willy, because Roo travels constantly, backwards. But, Willy does too, except he goes forward for a while. Then backwards. Rather odd assignment, but it generated a few good laughs and what not.
We then started looking at limits and concepts. The Sum, Difference, Product, and Quotient between 2 different Limits. The rules are simple. You can get some examples from the site Mr. K is going to put in the side bar. Simply Calculus I think it was called?
And for homework, we have section 2.8 all odd, and 24, and 30.
Horray. My job is finished... I guess. Sorry for the shortness and cheapness that it may appear in. But, for the most part, if I ever needed pictures, it's all in Mr. K's slides. Except for the ones that Mrs. (forgot her name) drew on the board. Which I need to add into my Tuesday scribe. Soon...
Thursday scribe. Whoever doesn't mind. I'll pick in class. Seeing the reaction should be worth it. Cya all in... about 6 hours. It's 8am when I made this scribe and yesterday's. Haha.
Yesterday's scribe! (Oct. 9th)
Tuesday's class was quite informative. But, more or less a review of Friday's lesson. (Read Robert's scribe post). We started off the class with a bit of troubleshooting with the smart board, and then followed by the a review. Mr. K helped us understand the concept of f' and f''. By doing so, he walked back and forth in the room, accelerating and with constant velocity. We were then given a group worksheet, given f', how is Willy moving during the race. Before we could finish that, a fire drill started. And it was very cold out there. The homework for Tuesday was... the Willy and the Race worksheet, and 2.7, all odd (I think. Need to edit this later.) Next scribe?!. Me. Again. Doesn't even count as picking myself. It's just me doing it a 2nd time. See you on my next post... yeah.
Wednesday, October 10, 2007
Today's Slides: October 10
Here they are ...
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
Labels:
Derivative Functions,
Mr. Kuropatwa,
Slides
Tuesday, October 9, 2007
Today's Slides: October 9
Here they are ...
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
Labels:
Derivative Functions,
Mr. Kuropatwa,
Slides
Monday, October 8, 2007
2.6 Inflection Points and the Socond Derivative
Hey everyone! Well here is the scribe for Friday’s class. The class started off with a quiz. This took about half the class. Then we went into the lesson on inflection points and the second derivative. We only got an introduction to this because of the quiz. This is what we went through:
· F is a function and f’ is the derivative of that function. Since f’ is also a function, it too can have its own derivative. It would be f’’.
· Recall that the derivative of a function tells you weather a function is increasing or decreasing.
· When tangent slops are increasing the graph of f is concave up.
· When tangent slops are decreasing the graph of f is concave down.
· A point on the graph of a function where the curve changes concavity is called an inflection point.
Since our class only got an intro to the lesson, that's about all I can tell you guys. The rest of the lesson should be resumed on Tuesday.
See all of you in class tomorrow. BYE!
Oh yeah the next scribe is…VAN!
· F is a function and f’ is the derivative of that function. Since f’ is also a function, it too can have its own derivative. It would be f’’.
· Recall that the derivative of a function tells you weather a function is increasing or decreasing.
· When tangent slops are increasing the graph of f is concave up.
· When tangent slops are decreasing the graph of f is concave down.
· A point on the graph of a function where the curve changes concavity is called an inflection point.
Since our class only got an intro to the lesson, that's about all I can tell you guys. The rest of the lesson should be resumed on Tuesday.
See all of you in class tomorrow. BYE!
Oh yeah the next scribe is…VAN!
Thursday, October 4, 2007
Wednesday, October 3, 2007
Today's Slides: October 3
Here they are ...
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
Labels:
Derivative Functions,
Mr. Kuropatwa,
Slides
Tuesday, October 2, 2007
The Derivative Assignment
Sorry for the late scribe post, but I had a lot of homework last night. Well yesterdays class began as usual with Grey-M posting the word of the day on the board and everyone awaiting the explanation. Except today was different, we had a fire drill, so we had to leave class and return a few minutes later. Then class had begun, with Mr. K reviewing a little bit on derivatives, and had showed us that in order to find out the equation of a derivative, you sometimes have to do some algebraic massage. An example on how it may be used are in the 3 slides Mr. K published yesterday, which explains the multiple steps needed.
Mr. K then gave out an assignment on the many different derivative questions which was done in groups for the rest of the class. The assignment explains how derivatives can be explained by a graph, a table, or an equation. This derivative assignment is due Wednesday. Also Mr. K assigned section 2.3 ( question #: All odd and #12) due for Wednesday as well. Tomorrow's scribe will be Sandy, and do not give the scribe duty to Grey-M as he has no Internet right now.

Mr. K then gave out an assignment on the many different derivative questions which was done in groups for the rest of the class. The assignment explains how derivatives can be explained by a graph, a table, or an equation. This derivative assignment is due Wednesday. Also Mr. K assigned section 2.3 ( question #: All odd and #12) due for Wednesday as well. Tomorrow's scribe will be Sandy, and do not give the scribe duty to Grey-M as he has no Internet right now.

Monday, October 1, 2007
Today's Slides: October 1
Here they are ...
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
To see a larger image of the slides go here. When you get there you'll see a button in the bottom right-hand corner that says [full]. Click it and the slides will display in full screen mode.
Labels:
Derivative Functions,
Mr. Kuropatwa,
Slides
Subscribe to:
Comments (Atom)








