Scribe (O.o)

Hello, I'm Tim-Math-y and I will be your scribe for today's class lessons. Today, we started off class by going over the requirements for scribe posts.

Remember that you are required to label your posts accordingly, especially your scribe posts. You are required to label your scribes with your name, unit, and most importantly, 'scribe'. Without labelling your scribe with 'scribe' is equivalent to not placing your name on your work; you will not receive credit due to completion on the scribelist (If you have not already fixed your labels, you should as soon as possible).

Next, we talked about del.icio.us accounts. If you have not already signed up for one such account, it is required as soon as possible. Remember to check out other tags in math, calculus, and the such for sites that other people found extremely useful. These sites will be helpful in contributing aid in our learning outside of the class.

After the brief run overs of these topics, we continued onto learning MATH! Today, we had a workshop to prepare us for a Pretest tomorrow and a Test on Thursday.

Workshop

1.a) For this question, we simply solved the lower and upper estimates using our calculators. We placed the equation f(x) = 4x - x^2 into y1 and used our RIESUM program to solve from the interval of [0,1]. Our solutions were L(4) = 1.28125 and U(4) = 2.03125.

1.b) We simply subtract the lower estimate from the upper estimate.

1.c) Here, we sketched 4 sub intervals on the interval of [0,1] of f(x). Here we geometrically sketched the upper and lower estimates to represent U(4) - L(4). The left sketch is incorrectly scaled whereas the right sketch is a correct drawing.

1.d) We used the equation: Error = |f(b) - f(a)| x ((b-a)/n) to solve. Here, 'b' is equal to 1 where 'a' is equal to 0.

2.) Here, we analysed the table to find out how we could estimate the integral on the interval of [-2,4]. Considering the fact that we need 3 sub intervals, we found that from (-2) -> (4) was 6 and so we could easily divide the information into 3 sub intervals. Here, we found the midpoint of 1.98 and 2.04 which was 1.03, midpoint of 2.04 and 9.64 which was 5.74, and the midpoint of 9.64 and 26.29 which was 16.82. We then added the three numbers and multiplied the sum by 2, because the subintervals maintained the length of 2. We found the result of 47.18 (Credit goes to Craig's group)

Another group attempted something different. They plotted the points onto the statplot using their calculators and simulated a similar quadratic graph. The solution was fairly close (46.5248) however despite the 99% accuracy, the answer was incorrect as it was not as accurate using the information given.

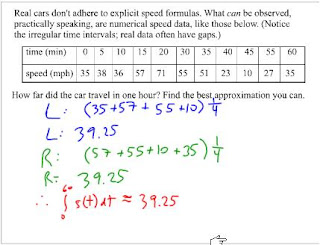3.) Here, Chris' group attempted to solve the problem. He came up with the idea to use four subintervals, seeing that the information given could not be broken up properly into 5s nor 10s and so he broke them into groups of 15s. This brought forth much sense. However, the answer was not the best approximation as he did not use all the information given.


Here, Craig's group again brought forth a solution. They used the RIESUM formula to find the best approximations on seperate intervals (the riesum finds the sum of the lower and upper estimates and divides it by 2). The intervals were: [0,20] which had intervals of 5 minutes, [20,30] which had an interval of 10 minutes, [30,45] which had intervals of 5 minutes, [45,55] which had an interval of 10 minutes, and [55,60] which had an interval of 5 minutes.

Adding together all the solutions, he found a more accurate approximation because he used all of the information given, despite the extreme excess of work compared to Chris' method.

End of Workshop

Word of the Day: Ginkgo - a herbal remedy derived from Jap/Chi tree to improve mental function and circulation.

Tomorrow's Scribe: aichelle s. Show them a nice scribe =)

Reminders: Tomorrow Pretest, Workshop Wednesday, Test Thursday

Have a great night everyone! Good luck on the pretest and test this week! Don't forget to BOB also! Good night!